List of formulae
Terms, definitions
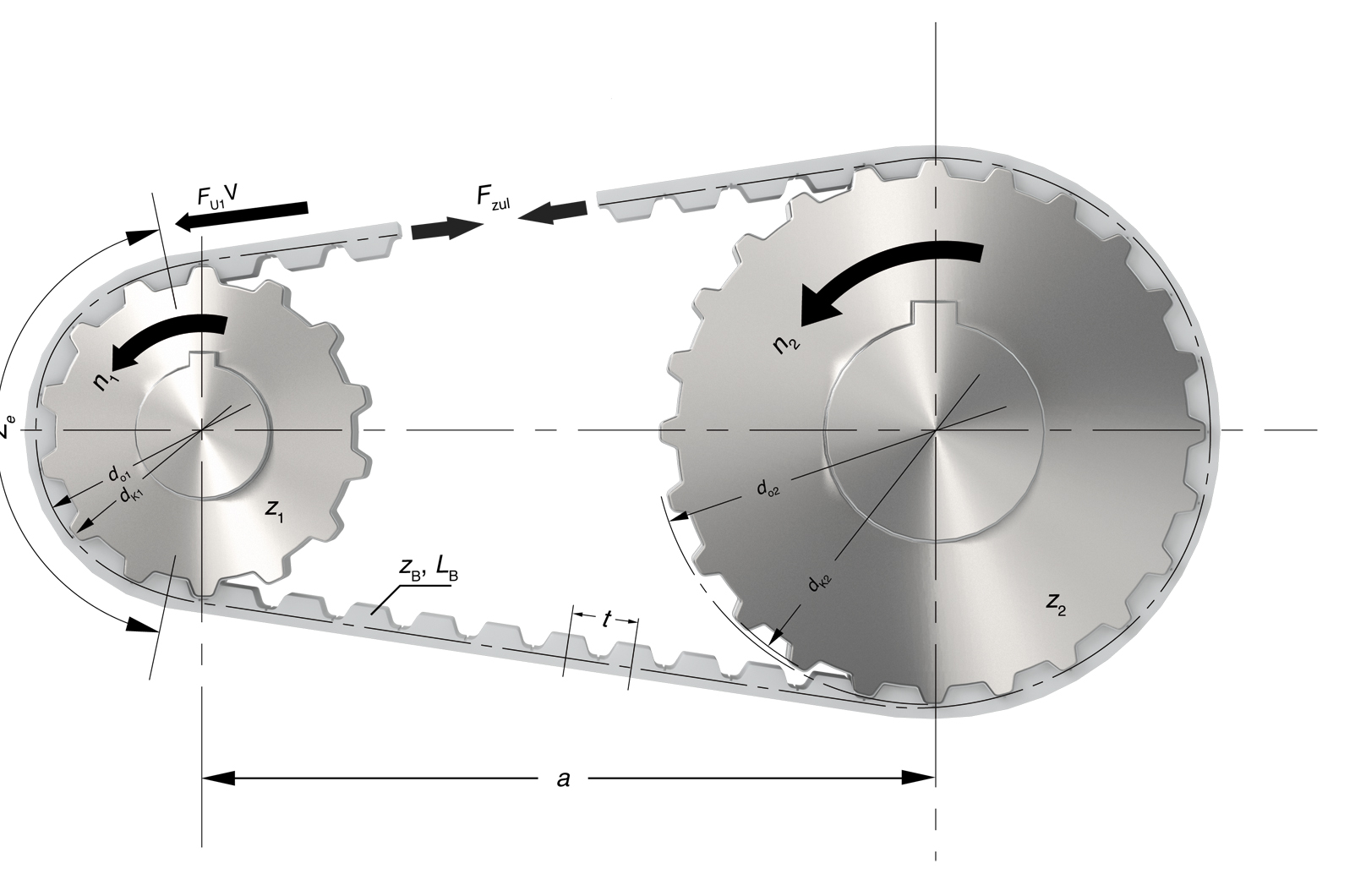
| Circumferential force | Fu | [N] | Centre distance | a | [mm] | |
| specific tooth force | FUspez | [N/cm] | Belt length | LB | [mm] | |
| admissible tensile force | Fzul | [N] | Belt width | b | [mm] | |
| Pre-tension force | Fv | [N] | Pulley width | B | [mm] | |
| Shaft force | Fw | [N] | Bore, pulley | d | [mm] | |
| Torque | M | [Nm] | Pitch circle diameter | d0 | [mm] | |
| Accelleration torque | MB | [Nm] | Crown diameter | dK | [mm] | |
| specific torque | Mspez | [Ncm/cm] | Span length | LT | [mm] | |
| Power | P | [kW] | Pitch | t | [mm] | |
| specific power | Pspez | [W/cm] | Number of belt teeth | zB | ||
| Load bearing torque | J | [kgm²] | Number of teeth with i=1 | z | ||
| Load | m | [kg] | Number of teeth in mesh | ze | ||
| Density | ρ | [kg/dm³] | No. of teeth, small pulley | z1 | ||
| Speed | v | [m/s] | No. of teeth, large pulley | z2 | ||
| Rotational speed | n | [min-1] | Transmission | i | ||
| Angular speed | ω | [s-1] | Accelleration time | tB | [s] | |
| Frequency | fe | [s-1] |
\begin{align} F_U & = {2 \cdot 10^3 \cdot M \over d_0} \\ & ={19,1 \cdot 10^6 \cdot P \over n \cdot d_0} \\ & ={10^3 \cdot P \over v} \end{align}
Circumferencial force
\begin{align} M & ={d_0 \cdot F_U \over 2 \cdot 10^3} \\ & ={9,55 \cdot 10^3 \cdot P \over n} \\ & ={d_0 \cdot P \over 2 \cdot v} \end{align}
Torque
\begin{align} P & ={M \cdot n \cdot F_U \over 9,55 \cdot 10^3} \\ & ={F_U \cdot d_0 \cdot n \over 19,1 \cdot 10^6} \\ & ={F_U \cdot v \over 10^3} \end{align}
Power
\begin{align} L_B & = 2a + \pi \cdot d_0 \\ & = 2a + z \cdot t \end{align}
Belt Length for i=1
\[d_0={z \cdot t \over \pi }\]
Pitch circle diameter
\[\omega={\pi \cdot n \over 30}\]
Angular speed
\[n = {19,1 \cdot 10^3 \cdot v \over d_0}\]
Rotational speed
\[v={d_0 \cdot n \over 19,1 \cdot 10^3 }\]
Peripheral speed
\[M_B={J \cdot \Delta n \over 9,55 \cdot t_B}\]
Acceleration torque
\[J={98,2 \cdot 10^{-15} \cdot B \cdot \rho \cdot (d_k^4 - d^4) }\]
Load bearing torque
Apply all equations with the dimensions mentioned here.